Interest Rate Options vs. FRAs

CFA Level 1 - Derivatives

A forward rate agreement (FRA) is an agreement between two parties to exchange a fixed interest payment for a floating interest payment. FRAs are OTC derivatives - forward contracts in which one party (which is referred to as the borrow or buyer) pays a fixed interest rate, and another party receives a floating interest rate equal to a reference rate (the underlying rate). The receiver is also referred to as the lender or seller.  The payments are calculated over a notional amount over a certain period and netted - in other words, only the differential is paid on the termination date.

Interest rate options give buyers the right, but not the obligation, to synthetically pay (in the case of a cap) or receive (in the case of a floor) a predetermined interest rate (the strike price) over an agreed period.

Similarities

• Both Interest rate options and FRAs have interest rates as their underlyer. 
• Both use put or call formats. 
• Both use a notional amount to define the size of the trade.
• Neither requires an exchange of principal.

Differences

• An FRA is a commitment to make one interest rate payment and receive another one at a future date while an option is the right to make one interest rate payment and receive another one.
• Interest rate options have exercise rate or strike rate instead of an exercise price like an FRA.

Option Payoffs
Payoffs for interest rate options function are similar to other options. The main difference is that the interest rate options take the days to maturity attached to the agreement into account. Also, the payoff from the option is not made until the end of the number of days attached to the rate. For example, if an interest rate option expires in 60 days and is based on 180-day LIBOR, the holder will not be paid for 180 days.

Interest rate call option payoffs are determined by the following formula:

Formula 15.3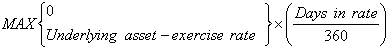

Interest rate put option payoffs are determined by the following formula:

Formula 15.4

Note that in each of the formulas above, the result of the equation is multiplied by the notional amount.

| | Print This! | No comments

Do you like this post?